Monday, September 13, 2021

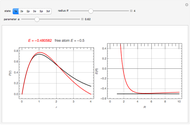
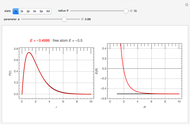
Eigenstates for a Hydrogen Atom Confined to an Infinite Spherical Potential Well

 

Download to Desktop

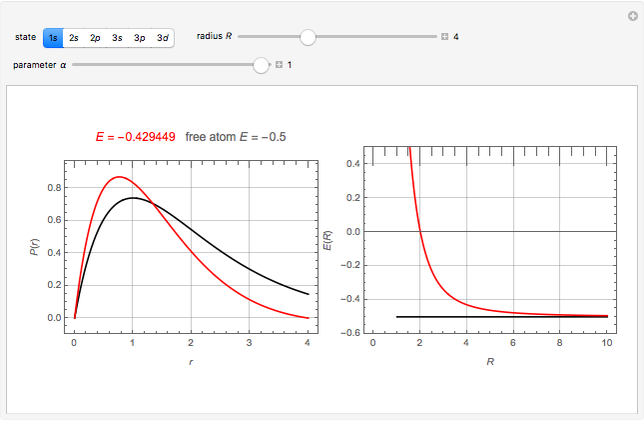
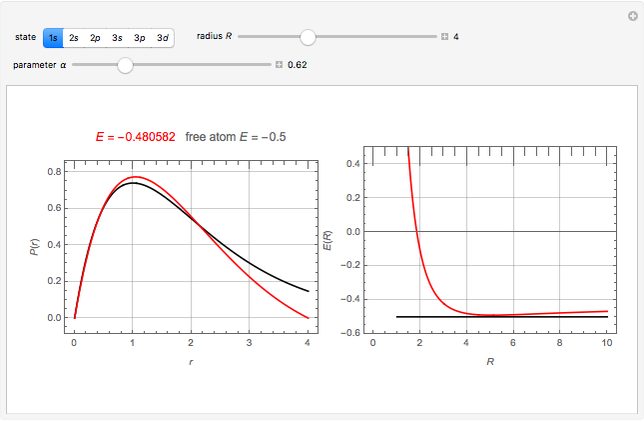
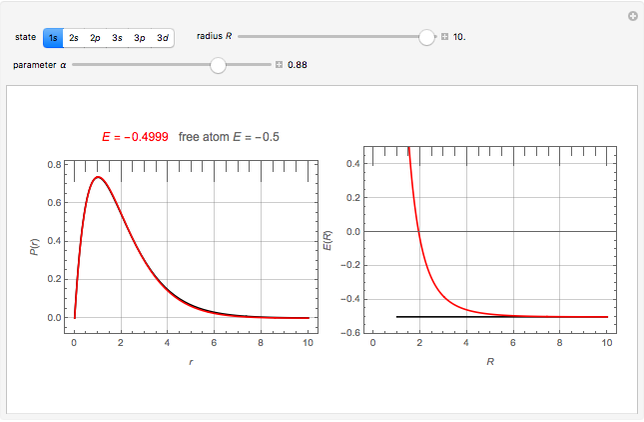
Apart from its intrinsic interest, the problem of a confined hydrogen atom was motivated by an attempt to model the effects of high pressure on a physical system. It is also relevant to quantum dots and white dwarfs. Among the many approaches to the problem have been numerical solutions, perturbation calculations and variational methods [1–3].

[more]

Contributed by: S. M. Blinder (November 2018)
Open content licensed under CC BY-NC-SA

Details

References

[1] C. Laughlin, B. L. Burrows and M. Cohen, "A Hydrogen-Like Atom Confined within an Impenetrable Spherical Box," Journal of Physics B: Atomic, Molecular and Optical Physics, 35(3), 2002 pp. 701–715. doi:10.1088/0953-4075/35/3/320.

[2] ‪S. H. Patil and Y. P. Varshni‬, "Properties of Confined Hydrogen and Helium Atoms," Advances in Quantum Chemistry, Vol. 57 (J. Sabin and E. Brandas, eds.), Amsterdam: Elsevier Academic Press, 2009 pp. 1–24 and references cited therein. doi:10.1016/S0065-3276(09)00605-4.

[3] S. H. Patil, "Wavefunctions for the Confined Hydrogen Atom Based on Coalescence and Inflexion Properties," Journal of Physics B: Atomic, Molecular and Optical Physics, 35(2), 2002 pp. 255–266. stacks.iop.org/0953-4075/35/i=2/a=305.

Snapshots

 

 https://demonstrations.wolfram.com/EigenstatesForAHydrogenAtomConfinedToAnInfiniteSphericalPote/

Posted by at
Labels:

No comments:

Post a Comment

Subscribe to: Post Comments (Atom)