Relativistic Energy Levels for Hydrogen Atom
| |||
In nonrelativistic quantum mechanics, the energy levels of the hydrogen atom are given by the formula of Bohr and Schrödinger, 
,
expressed in hartrees (assuming the appropriate correction for the
reduced mass of the electron). The energy depends only on the principal
quantum number 
and is 
-fold
degenerate (including electron spin). In Dirac's relativistic theory,
this degeneracy is partially resolved and the energy is found to depend
as well on the angular-momentum quantum number 
. To second order in the fine-structure constant 
, the hydrogen energy levels are given by 
. In Dirac's theory, levels such as 
and 
remain degenerate. The discovery of the Lamb shift showed that these
two levels were actually split by 1057.8 MHz. This was a major stimulus
for the development of quantum electrodynamics in the 1950s. The Lamb
shift, significant only for 
-states), raises the energy by approximately 
. The relativistic and radiative correction to hydrogen energy levels can therefore be written 
, to third order in 
.
In this Demonstration, you can conceptually vary the fine-structure
constant from 0 to its actual value, or equivalently the speed of light 
from 
to 1 (meaning 
m/s), to show the transition from nonrelativistic to relativistic energies for quantum numbers 
, and 
. The energies are expressed in MHz (1 hartree = 
MHz).
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
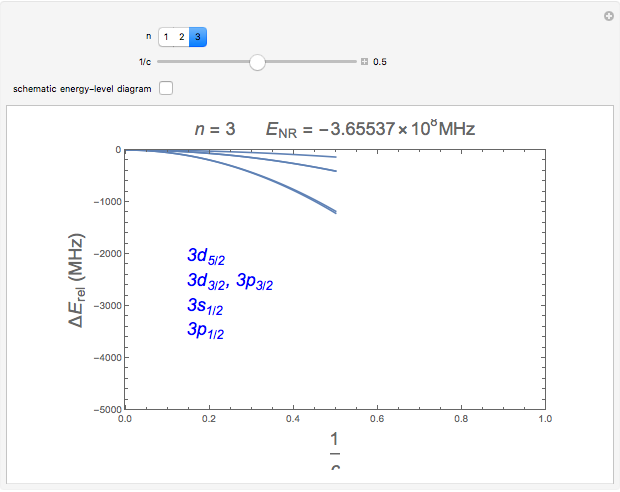
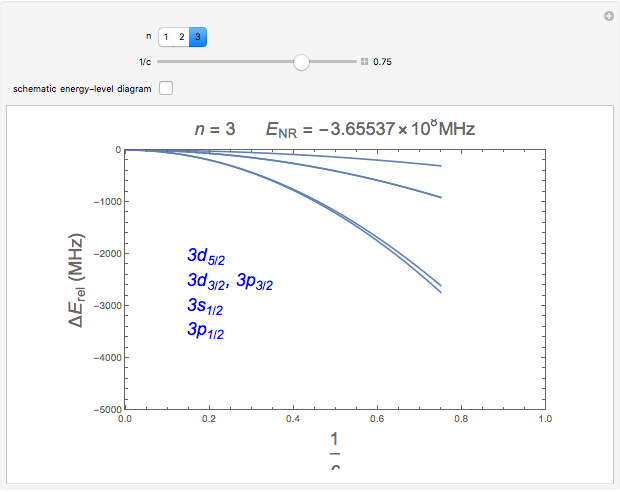
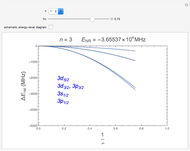
Snapshots 1, 2: relative corrections to 
level as speed of light is conceptually decreased from infinity
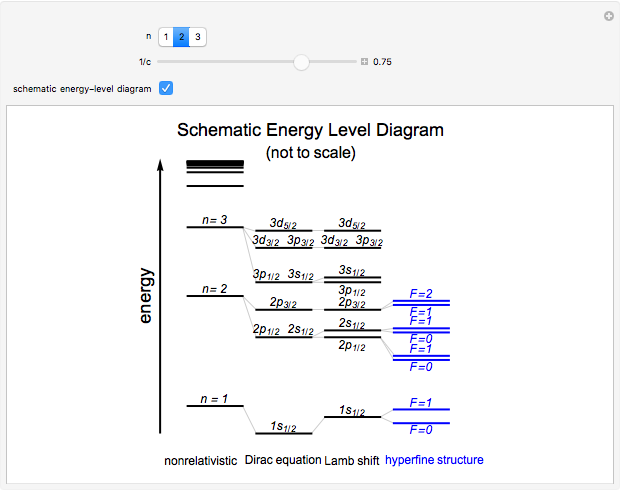
Snapshot 3: schematic hydrogen energy-level diagram for various stages of theory
Permanent Citation
https://demonstrations.wolfram.com/RelativisticEnergyLevelsForHydrogenAtom/
No comments:
Post a Comment