The Hydrogen Atom in Parabolic Coordinates

The Schrödinger equation for the hydrogen atom,  (in atomic units
(in atomic units  ), can be separated and solved in parabolic coordinates
), can be separated and solved in parabolic coordinates  as well as in the more conventional spherical polar coordinates
as well as in the more conventional spherical polar coordinates  .
This is an indication of degeneracy in higher eigenstates and is
connected to the existence of a "hidden symmetry", namely the
.
This is an indication of degeneracy in higher eigenstates and is
connected to the existence of a "hidden symmetry", namely the  Lie algebra associated with the Coulomb problem. Parabolic coordinates can be defined by
Lie algebra associated with the Coulomb problem. Parabolic coordinates can be defined by  ,
,  , with the same
, with the same  as in spherical coordinates. The wavefunction is separable in the form
as in spherical coordinates. The wavefunction is separable in the form  with
with  . Here
. Here  is a Whittaker function and
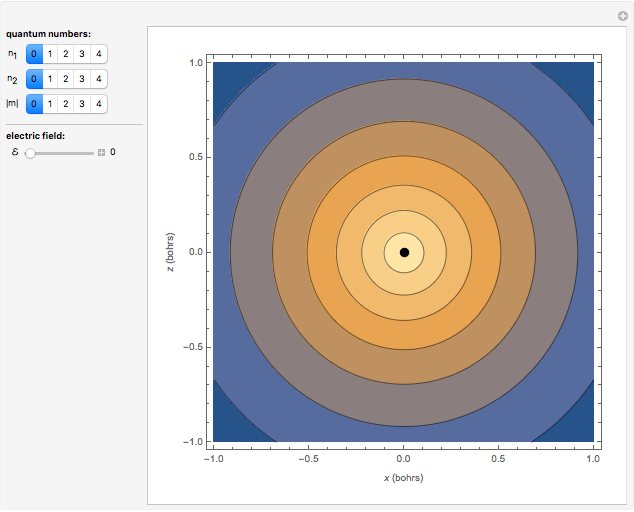
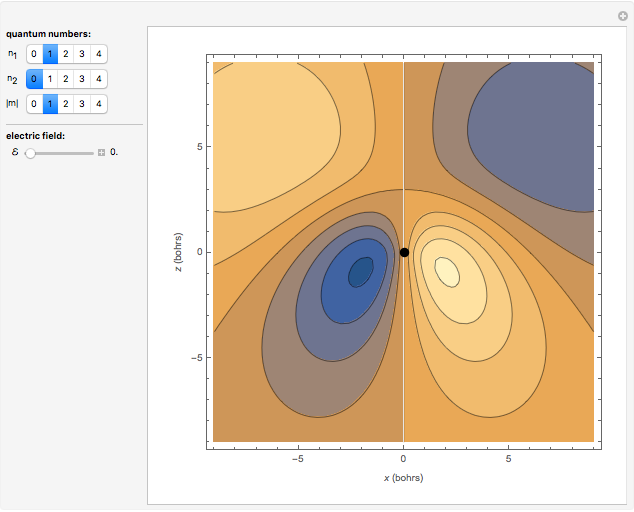
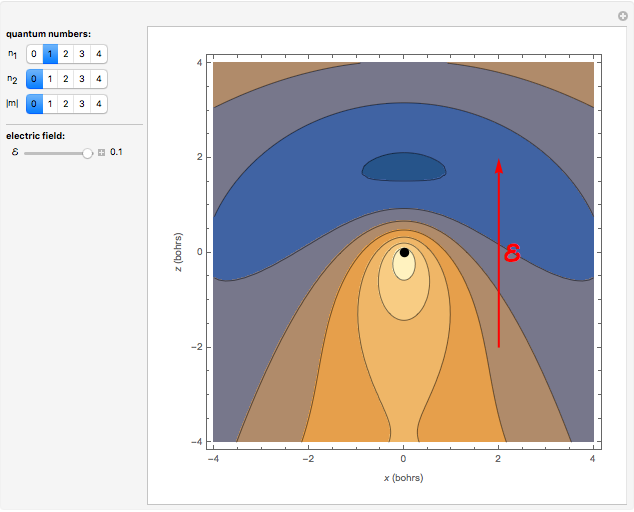
is a Whittaker function and  , equal to the principal quantum number. Contour plots for the real part of the wavefunctions in the
, equal to the principal quantum number. Contour plots for the real part of the wavefunctions in the  -plane are shown, including the values
-plane are shown, including the values  and
and  . The nucleus is represented as a black dot. The corresponding energy eigenvalues are given by
. The nucleus is represented as a black dot. The corresponding energy eigenvalues are given by  , independent of other quantum numbers (in the field-free nonrelativistic case).
, independent of other quantum numbers (in the field-free nonrelativistic case).
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Snapshot 1: the  ground state
ground state
Snapshot 2: a  -
- hybrid atomic orbital
hybrid atomic orbital
Snapshot 3:  state in an electric field
state in an electric field
Reference: H. Bethe and E. R. Salpeter, Quantum Mechanics of One- and Two-Electron Atoms, New York: Academic Press, 1957 pp. 27–29 and 228–234.
Permanent Citation
https://demonstrations.wolfram.com/TheHydrogenAtomInParabolicCoordinates/



No comments:
Post a Comment